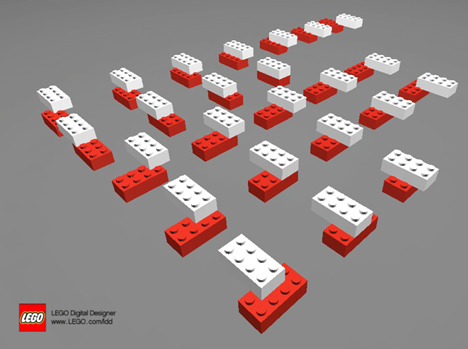
Did you know that you can combine two identical 2×4 LEGO ® bricks 24 different ways? Of course you did. That fact has been plastered just about everywhere LEGO bricks can be found. But have you ever really counted for yourself?
Desperate for something mathematical to write about and having come across this fact yet again in the LEGO Catalog from Toy Fair, I decide to enumerate them for myself. Here is how you can do it.
First, get yourself two identical 2×4 LEGO bricks. I recommend red. I used one white brick in the above picture though so you could more easily see how they connect.
Place one brick in your left hand so it is twice as tall as it is wide, and place the other brick in your right hand the same way. Now, connect the brick in your right hand to the one in your left hand such that the top-left corner of the right-hand brick is on top of the bottom-right corner of the left-hand brick. That is position number one. You can see it in the top-right corner of the above picture.
Positions two through seven, the rest of the top row, are discovered by “walking” the top brick one stud at a time up along the right side of the bottom brick. Position two has two studs connected, three has three studs, four has four studs, five has three studs, six has two studs, and seven has one stud.
Positions eight through eleven, the next row down, are exactly like positions one through four, except this time we line up the two bricks and connect two studs to two studs, four studs to four studs, six studs to six studs, and eight studs to eight studs.
Why don’t we keep on going for positions twelve through fourteen like we did with five through seven? Because of symmetry. If you were to keep on going, then what you would think would be position twelve is actually position ten, rotated 180 degrees.
That same symmetry is going to keep us from moving over one more stud and running up the left side the way we ran up the right. Connecting to the left side is exactly the same as connecting to the right side in mirror image and rotating 180 degrees.
So where do the other thirteen positions come from? They come from rotating our left-hand (bottom) brick 90 degrees so it is now twice as wide as it is tall and perpendicular to our right-hand (top) brick.
There are five positions (numbers twelve through sixteen, in the middle row) where we can run the left-hand side of the right-hand brick along the right-hand side of the left-hand brick. We can repeat the proceedure again, but this time using the full width of the right-hand brick, along the right-hand side of the left hand brick, for another five positions (numbers seventeen through twenty-one, in the fourth row).
Position twenty-two connects the top of the right-hand brick to the middle of the left-hand brick. Position twenty-three advances that forward, so the top-half of the right-hand brick covers the middle of the left-hand brick. And finally, position twenty-four has the two middles of the two bricks connected together to form a + (cross or plus).
You could continue to advance the right-hand brick to create other positions, but once again symmetry comes into play and all subsequent positions are but rotations of earlier enumerated positions.
0 Comments